Mathematics Weblog
Powers
Friday 25 June 2004 at 3:37 pm | In Articles | 3 CommentsI was asked recently why  . Remember that if n is a positive whole number then
. Remember that if n is a positive whole number then 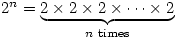 . Clearly you can’t multiply 2 by itself 0 times 😕
. Clearly you can’t multiply 2 by itself 0 times 😕
The key, when extending properties of the number system, is to use definitions that work for every number. So, for example
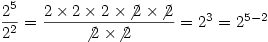
which gives you the rule that
- to divide powers you subtract the indices (the small superscripted numbers)
This leads to
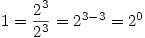
Similarly, 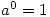 for any positive real number.
for any positive real number.
What about zero powers of non-negative powers?  is a controversial case I have mentioned on 29 February (Q2.) and see Dr Math FAQ for more on this.
is a controversial case I have mentioned on 29 February (Q2.) and see Dr Math FAQ for more on this.
And if the number is negative? Great care is needed in this case. For example, using only real numbers, 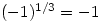 but
but 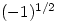 is not a real number. The problem arises because the general definition of a power is given by
is not a real number. The problem arises because the general definition of a power is given by 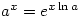 and
and 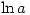 is undefined if a is negative or 0. Using complex numbers (which helps with
is undefined if a is negative or 0. Using complex numbers (which helps with 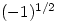 ) just makes things more complex 😕 – see Log of Complex Number
) just makes things more complex 😕 – see Log of Complex Number
Misunderstanding
Wednesday 16 June 2004 at 4:23 pm | In Articles | 1 CommentWhen teaching maths that you are familiar with, it is not easy to see why students struggle with it – indeed once the student has understood the problem, they can’t see why they had difficulty before! This means that you have to be very careful what you say in case an attentive student takes it literally.
How many times has a lecturer said “the integral of e is itself ” ? So this happens:
- Q. Find the value of
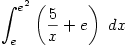
A. ![\displaystyle \int_e^{e^2}\left(\frac{5}{x}+e\right)\ dx=\left[5\ln |x| + e\right]_e^{e^2}=\dots=5 \displaystyle \int_e^{e^2}\left(\frac{5}{x}+e\right)\ dx=\left[5\ln |x| + e\right]_e^{e^2}=\dots=5](/maths/latexrender/pictures/e846d6c1a3346703ff51cd012cc2f2bb.gif)
When the error was pointed out to the student, they responded with
- Why does
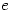 integrate to
integrate to 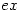 ?
? 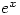 integrates to
integrates to 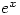 and as
and as 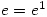 surely the integral of
surely the integral of 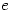 is also
is also 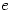 ?
?How would you respond to this?
Generalisation of derivative
Sunday 6 June 2004 at 2:25 pm | In Articles | Post CommentInspired by a posting on S.O.S. Mathematics CyberBoard
Most students will be familiar with the definition of the derivative of a real-valued function of a real variable defined on some interval (a,b):
- If
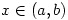 then f is differentiable at
then f is differentiable at 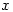 if
if 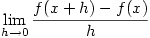 exists and the limit is denoted
exists and the limit is denoted 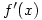
It is also clear that for this to make sense  must be defined at
must be defined at 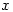 (and of course it is a well-known consequence of the definition that
(and of course it is a well-known consequence of the definition that  is also continuous at
is also continuous at 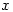 ). But what if
). But what if  is defined on
is defined on 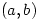 but not at
but not at 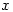 , can we do anything then? Yes, we can define a pseudo-derivative
, can we do anything then? Yes, we can define a pseudo-derivative 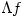 of
of  provided
provided  is defined on a neighbourhood of
is defined on a neighbourhood of 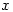 :
:
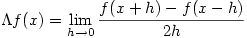
This pseudo-derivative has similar properties to the derivative and indeed it has the same values where  is differentiable but there are significant differences as the following exercises show:
is differentiable but there are significant differences as the following exercises show:
- If
 is differentiable at
is differentiable at 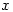 show that
show that 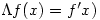
- If
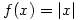 show that
show that 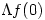 exists although
exists although 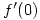 does not
does not - If
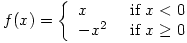 show that
show that  has a local maximum at 0 but
has a local maximum at 0 but 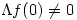
- Suppose
 is differentiable on
is differentiable on 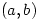 , except at a point
, except at a point 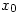 in
in 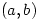 , with
, with 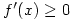 for
for 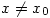 .
.
If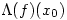 exists show that
exists show that 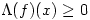
Powered by WordPress with Pool theme design by Borja Fernandez.
Entries and comments feeds.
Valid XHTML and CSS. ^Top^