Mathematics Weblog

Oh dear!

At the risk of repeating myself, I just have to post some questions a student appears to have been asked to answer by his teacher. I say appears because the student was seeking help and may have misunderstood the questions, but I doubt it. They are horrifying.

In the following questions, say which of

a) 0    b) 1    c)    (d) indeterminate

is the correct answer:

1.	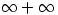5.	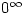9.	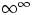13.
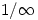2.	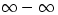6.	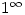10.	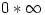14.
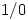3.	7.	11.	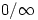15.
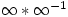4.	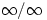8.	12.	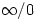16.

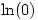Yes the questions do purport to be about the normal real number system. Shocking, isn’t it, that a student could be asked such drivel? 🙁

PS Just come across Not Infinity! which gives a good explanation of this type of misuse.

Wonderful quote

“In fact that is one of the first things we beat out of students – misapplications of equals signs, until such time as they are sufficiently well read to start abusing them again.”
matt grime at SFN

Mathematics lecturers everywhere will be nodding in agreement 😎

What does 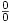mean?

I have written about the nonsense that comes about when you divide by 0 or attempt to use like a number. So I was delighted to come across John Conway’s comments at MathPath. John Conway is of course one of the best mathematicians today, having, amongst many other things, invented the Game of Life and discovered three sporadic finite simple groups, known as the Conway groups

Nice Problem

Here’s a nice problem taken from ChapterZero who, in turn, got it from Rudin’s Principles of Mathematical Analysis

Given the sequence defined as 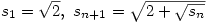, show that converges, and that it is bounded above by 2.

More maths blogs?

I’ve mentioned before about a lack of mathematics blogs. Now the mathematics site Art Of Problem Solving is creating a list of blogs of members of its community.

OK, so the blogs may not be strictly mathematics blogs (mathematicians do have other interests believe it or not) but they could well be of interest to other mathematicians. Keep an eye on the blog list!

PS See also the list of Maths Blogs on the right (which is recursive 8-))

Writing Mathematics

Isabel’s math blog is one of those rare things – a mathematics blog; let’s hope it will inspire others. Isabel is a fourth-year undergraduate mathematics student at MIT who has been marking first-year students’ homework. She makes some excellent points that are relevant at all levels including A-level.

I hope she won’t mind me quoting some of what she says at on grading homework:

• If the problem calls for some numerical answer, or for some symbolic expression as an answer, and the student produces a wrong answer, they can’t get full credit. (Very near full credit, say nine out of ten, is possible.)
• Not surprisingly, if you know what the answer to a problem is supposed to be, it’s easy to make errors that “cancel each other out”; if you know where the target is you’ll make some pretty strange logical leaps to get there. (I’ve been guilty of this too. Potential approaches to a problem that seemed incredibly stupid at noon the day before the homework is due seem quite reasonable at three in the morning.)
• The question is, how does one teach students “clarity” in mathematics? One of the things I notice is that the students don’t realize the power of the English language. They write down endless strings of equations, and make no effort to connect these with words that explain what they’re doing. Fortunately for them, as the grader I know what they’re trying to do. But at the same time, sometimes I don’t know what they’re trying to do; they manage to confuse me quite thoroughly.
  There seems to be a flaw in mathematics education – that we don’t teach students how to write mathematics.

My thoughts exactly 🙂