Equation Wizard

Sunday 29 April 2007 at 5:43 pm | In Articles | 7 Comments

I have an ambivalent attitude to mathematical software. On the one hand, an enthusiastic user of LaTeX both here and in documents and presentations, but, on the other hand, wary of too much reliance on calculators and computers. One superb maths teacher I know was criticised for not using a computer in his A level class. A Chinese student in the class remarked that they didn’t see much benefit in using one and they had only started to use a calculator when they came to this country to study A levels. They have an important point; I recoil when I ask a simple arithmetic question and the student instinctively reaches for their calculator. Worse still is when they use the calculator on their phone and don’t believe me when I tell them the calculator gets it wrong because it doesn’t obey basic mathematical rules. Similarly, I find graphics calculators are too complicated (computer programs are much easier to use to sketch graphs) and I would prefer to teach students how to sketch curves so that they get a feel for the properties of various functions.

Of course there is a place for calculators and mathematical software. Checking my tax would be a pain without a calculator and graphing software for focusing in at what happens near the origin of the graph of functions like
f(x)= \begin{cases} x^n\sin\left(\frac{1}{x}\right) & x\neq 0 \\ 0 & x=0 \end{cases}
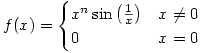
is fascinating. Similarly, if I am marking student work I use mathematical software to check their matrix operations rather than doing them by hand. Some mathematical software is very powerful and expensive but there are free sites like QuickMath which will solve many problems. However, they should really be used to save time or offer insights after the techniques have been taught and understood – practice, practice and practice is often the best way to learn.

So when I was asked by ElasticLogic to review their Equation Wizard  I made it clear that I would be offering an honest opinion of the program that they sent me.

Equation Wizard is a Windows only program that solves real rational equations and simplifies rational expressions (rational means ratios of polynomials). In fact it claims to solve algebraic equations but that is a misnomer as it doesn’t solve equations involving fractional powers or complex coefficients, though it will give some complex roots (for some reason called imaginary roots in Help). QuickMath does this for free but Equation Wizard’s strength comes from the fact that it will show the working so the user can understand the method behind the solution.

Entry of polynomials is easy using ^ for powers or using buttons or menus or the Ctrl key and the text is previewed in mathematical form as you type, so x^2 becomes 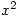x^2 and (x^2-1)/2 becomes 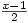\frac{x-1}{2}; the previewer does its best to interpret ambiguous expressions such as 1/2x. It will add algebraic fractions showing the working, so if you input 1/(x-1)+1/(x+1) then it is simplified to 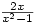\frac{2x}{x^2-1} by adding using a common denominator, multiplying out and collecting the terms in the numerator to get the result, with all steps shown. It uses a similar method to solve the equation 1/(x+1)+1(x+1)=1 finding the answer to 3 decimal places (or up to 9 decimal places if required). There appears to be no limit to the degree of the polynomial equations to be solved – solving 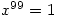x^{99}=1 was virtually instant.

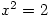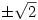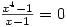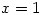However, there are limitations. The answers given cannot give exact values so x^2=2 gives 1.414 and -1.414 rather than \pm\sqrt{2}. Rational equations are solved by multiplying by the denominator but the solutions aren’t checked so \frac{x^4-1}{x-1}=0 gives x=1 as one of four solutions. My Norwegian students were taught to always check their answers, so would know what to do. On the other hand solutions can be missed so 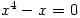x^4-x=0 gives 0 and 0 as the two solutions, though I expect that’s just a bug. However, 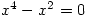x^4-x^2=0 gives one correct answer and two wrong ones, which is rather worrying. There are language problems with 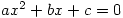ax^2+bx+c=0 being described as a quadric equation. Quadric surfaces are interesting and there are nice pictures here.

You can print out the calculations or save them in rtf or HTML format (with the equations saved as images) but it would be nice to be able to copy the selected output to the clipboard. Having solved an equation it’s not possible to change it – you have to enter a new one, though you can copy and paste the old one or use the history button, bizarrely labelled \Sigma.

Your opinions on Equation Wizard are welcome and I hope ElasticLogic will improve this early version as a result of feedback from you. Can you find other equations with missing or incorrect solutions? The software costs $29 (or £15.55 in real money 🙂 ) and you can download a free trial version here.

Mathematics in the News

Tuesday 24 April 2007 at 7:11 pm | In Articles | 11 Comments

Today the BBC reports two stories about mathematics

“I did maths at school and for one year at university but I don’t think I was ever very good at it – and some people would say it shows,” Mr Brown laughed.

I wish he hadn’t laughed (was that due to guilt?) but in his defence he has probably studied mathematics to a higher level than most politicians. It should also be noted that he was educated in Scotland where the first year of university is the equivalent to the last year of school in England and Wales (and so is more like the US system). So he is likely to have studied mathematics to A level standard.

Pupils are being discouraged from taking A-level maths as schools in England chase higher places in the league tables, scientists have claimed.

The Royal Society of Chemistry said that as maths was a difficult subject, schools feared examination failures which would threaten their standings.

Of course the DES totally miss the point when they say

The Department for Education and Skills said more pupils were studying maths.

More than what? Such is the pressure of those league tables that I can totally believe this story. I wonder sometimes if we shouldn’t rename this country Wonderland and then find an Alice who can make sense of it all.

Dumbing Down 2

Saturday 14 April 2007 at 10:42 am | In Articles | 8 Comments

In Dumbing Down I mentioned the concern about mathematics education in Washington State USA. There is now a campaign there about ‘reform math’ which appears to be a system of education that tries to avoid teaching mathematics skills. As part of the campaign you can watch videos on YouTube from meteorologists Math Education: An Inconvenient Truth (which unfortunately stops half-way through) and Math Education: A University View. The latter video claims that California abandoned ‘reform math’ some years ago and as a result had an astonishing increase in students’ mathematical performances. You can read more on the campaign’s website Where’s the Math?

I shall be following this debate because, where the US goes, the UK often follows. This dumbing down of mathematical education may be linked to the shortage of good mathematics teachers since such ‘reform math’ can be taught by non-mathematicians. It’s clear that the descent into a downward spiral soon follows.

In the UK there has been a suggestion of ‘bribing’ students to study maths and science A levels ‘Pay students’ to revive science – are today’s students that shallow? How about good mathematics teaching which imbues a sense of wonder and delight?

 Alexandre Borovik in Division of Labour suggests that

The era of extensive mathematical education of majority is over — we have to develop a model of intensive mathematical education of minority.

He is not convinced that the mathematical education community can do this. I like the idea of extending the syllabus for those students who have the ability but I am also concerned about not leaving mathematics to an elite totally cut-off from the rest of the country.

 Hence my previous question in Dumbing Down

Is it possible to teach mathematics to a larger range of students without compromising on the level taught?

PS I just have to add this quote from The Unapologetic Mathematician discussing this issue because it encapsulates what I have been trying to say for years though I fear it fell on deaf ears

The algorithm comes first, and understanding comes later. Mathematics simply is. It cannot be negotiated. Mathematics education as realized in the NCTM standards has been taken over by sociologists, or even Critical Theorists. They are vehemently opposed to the seemingly-authoritarian rote method and saying “just do it like this and don’t ask why”. Never mind the fact that in this case “why” comes naturally after “how”. And it’s about time for mathematicians to come down and start kicking some ass over this, or we’ll be left with nobody capable of replacing us.

Another Chance to Read … Calendars

Monday 2 April 2007 at 1:56 pm | In Articles | 4 Comments

It’s not just television that thrives on repeats; I thought it worth repeating a posting about calendars from a year ago … 

It is this time of year that has attracted a lot of attention devoted to finding the dates of religious festivals. A very comprehensive calendar calculator for 25 different calendar systems can be found at Calendrica (Java applet) and details of these systems are given in a fascinating book Calendrical Calculations.

Algorithms to calculate Easter dates have been given by mathematicians through the ages, including Gauss (see for example Mind Over Mathematics: How Gauss Determined The Date of His Birth) but it does produce a few errors. In 1961 the Scottish mathematician T.H. O’Beirne published an algorithm in his Puzzles and Paradoxes column in the New Scientist subsequently reprinted in his book of the same name published by the Oxford University Press (sadly out of print but I throughly recommend looking for a second-hand copy).

O’Beirne’s algorithm (based on an 1876 article in Nature) has the merit of always giving the correct date as well as being easy to use. It is a simple exercise to write a program to do the work for you. Simple Example

O’Beirne’s algorithm
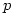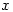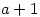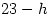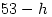The following process gives the date of Easter Sunday as the pth day of the nth month in year x. It also gives the Golden Number a+1 and the epact (23-h or 53-h whichever is between 1 and 30 inclusive). All you have to do is start with the year 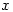x and perform 10 division operations noting the quotients and remainders.

\begin{tabular}{|c|l|r|c|c|} \cline{1-5} \multicolumn{1}{|l|}{Step} & Divide & By & Quotient & Remainder \\ \cline{1-5} 1 & $x$ & 100 & $b$ & $c$ \\ \cline{1-5} 2 & $5b+c$ & 19 & - & $a$ \\ \cline{1-5} 3 & $3(b+25)$ & 4 & $\delta$ & $\epsilon$ \\ \cline{1-5} 4 & $8(b+11)$ & 25 & $\gamma$ & - \\ \cline{1-5} 5 & $19a+\delta-\gamma$ & 30 & - & $h$ \\ \cline{1-5} 6 & $a+11h$ & 319 & $\mu$ & - \\ \cline{1-5} 7 & $60(5-\epsilon)+c$ & 4 & $j$ & $k$ \\ \cline{1-5} 8 & $2j-k-h+\mu$ & 7 & - & $\lambda$ \\ \cline{1-5} 9 & $h-\mu+\lambda+110$ & 30 & $n$ & $q$ \\ \cline{1-5} 10 & $q+5-n$ & 32 & 0 & $p$ \\ \cline{1-5} \end{tabular}
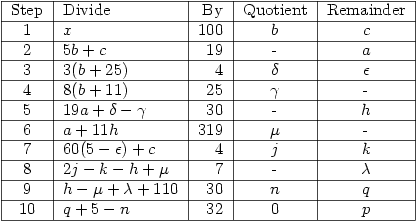
(Table produced by LaTable)

Powered by WordPress with Pool theme design by Borja Fernandez.
Entries and comments feeds. Valid XHTML and CSS. ^Top^