Mathematics Weblog
Stretching Question 2
Friday 13 October 2006 at 3:14 pm | In Articles | 3 CommentsIn Stretching Question I wondered how students would cope with a type of question they wouldn’t have seen before:
- If
 calculate
calculate  and
and 
The examiners’ report is now out and it says (edited to show context)
- Attempts at calculating
 were rarely completely successful. Many candidates found that
were rarely completely successful. Many candidates found that 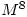 was the identity matrix but then went on to say that
was the identity matrix but then went on to say that  must also be the identity matrix. Others gave the value of
must also be the identity matrix. Others gave the value of 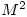 or gave the correct answer without any indication as to their reason for doing so, or with incomplete reasoning.
or gave the correct answer without any indication as to their reason for doing so, or with incomplete reasoning.How disappointing that so few managed to complete the question successfully. The lack of reasoning doesn’t tell us whether students guessed or found it too easy to bother putting down the working. It might have helped if students were taught that if 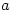 and
and 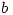 are integers then there exist integers
are integers then there exist integers 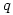 and
and 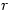 with
with 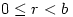 such that
such that 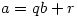 but these fundamental ideas seemed to have been lost to A level.
In the first AS exam the examiners comment that
but these fundamental ideas seemed to have been lost to A level.
In the first AS exam the examiners comment that
- When asked to use the Factor Theorem or Remainder Theorem, no marks can be earned for using long division.
which means
- British students haven’t a clue how to do long division of polynomials so we’ve made it easier for you, so don’t try and pretend you know best. (See A Level Exam Questions).
3 Comments »
RSS feed for comments on this post. TrackBack URI
Leave a comment
Powered by WordPress with Pool theme design by Borja Fernandez.
Entries and comments feeds.
Valid XHTML and CSS. ^Top^
the matrix looks like the rotational matrix for rotating a point 45 degrees. so since M is a rotation by 45 degress then M^n is a rotation by (45)(n) degrees.
Comment by nick james — Sunday 22 October 2006 8:45 am #
Good point especially as students were asked to identify the transformation that M represents. I wonder how many, if any, students used that when they found that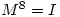 .
.
Whatever way is used, students still need to be somehow aware of the fact that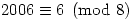
Comment by Steve — Sunday 22 October 2006 12:37 pm #
it is easy to see some cosinuses or sinuses there,so the problem is solved whereas
there are many trigonometric relations known
Comment by stefan petrea — Tuesday 31 October 2006 1:29 am #